Convex hull of zeros
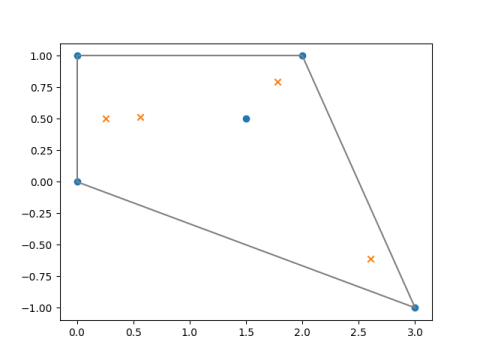
There's a well-known theorem in complex analysis that says that if p is a polynomial, then the zeros of its derivative p' lie inside the convex hull of the zeros of p. The convex hull of a set of points is the smallest convex set containing those points.
This post gives a brief illustration of the theorem. I created a polynomial with roots at 0, i, 2 + i, 3-i, and 1.5+0.5i. The convex hull of these points is the quadrilateral with corners at the first four roots; the fifth root is inside the convex hull of the other roots.
The roots are plotted with blue dots. The roots of the derivative are plotted with orange *'s.
In the special case of cubic polynomials, we can say a lot more about where the roots of the derivative lie. That is the topic of the next post.
More complex analysis postsThe post Convex hull of zeros first appeared on John D. Cook.