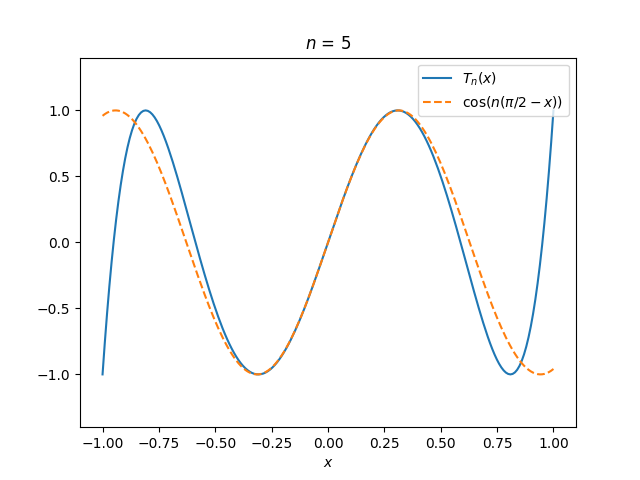
Chebyshev polynomials as distorted cosines

Forman Acton's book Numerical Methods that Work describes Chebyschev polynomials as

cosine curves with a somewhat disturbed horizontal scale, but the vertical scale has not been touched.

The relation between Chebyshev polynomials and cosines is

T_n(cos ) = cos(n).

Some sources take this as the definition of Chebyshev polynomials. Other sources define the polynomials differently and prove this equation as a theorem.

It follows that if we let x = cos then

T_n(x) = cos(n arccos x).

Now sin x = cos(/2 - x) and for small x, sin x x. This means

arccos(x) /2 - x

for x near 0, and so we should expect the approximation

T_n(x) cos(n(/2 - x)).

to be accurate near the middle of the interval [-1, 1] though not at the ends. A couple plots show that this is the case.

Mote Chebyshev posts

• Product of Chebyshev polynomials
• Chebyshev's other polynomials
• Chebyshev approximation

The post Chebyshev polynomials as distorted cosines first appeared on John D. Cook.