Cepstrum, quefrency, and pitch

John Tukey coined many terms that have passed into common use, such as bit (a shortening of binary digit) and software. Other terms he coined are well known within their niche: boxplot, ANOVA, rootogram, etc. Some of his terms, such as jackknife and vacuum cleaner, were not new words per se but common words he gave a technical meaning to.

Cepstrum is an anagram of spectrum. It involves an unusual use of power spectra, and is roughly analogous to making anagrams of a word. A related term, one we will get to shortly, is quefrency, an anagram of frequency. Some people pronounce the 'c' in cepstrum hard (like 'k') and some pronounce it soft (like 's').

Let's go back to an example from my post on guitar distortion. Here's a note played with a fairly large amount of distortion:

And here is its power spectrum:

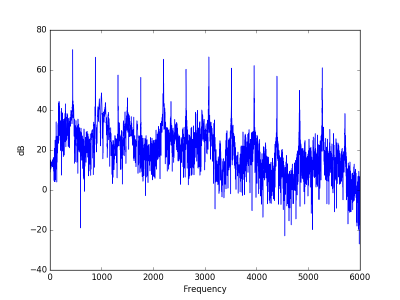
There's a lot going on in the spectrum, but the peaks are very regularly spaced. As I mentioned in the post on the sound of a leaf blower, this is the fingerprint of a sound with a definite pitch. Spikes in the spectrum alone don't indicate a definite pitch if they are irregularly spaced.

The peaks are fairly periodic. How to you find periodic patterns in a signal? Fourier transform! But if you simply take the Fourier transform of a Fourier transform, you essentially get the original signal back. The key to the cepstrum is to do something else between the two Fourier transforms.

The cepstrum starts by taking the Fourier transform, then the magnitude, then the logarithm, and then the inverse Fourier transform.

When we take the magnitude, we throw away phase information, which we don't need in this context. Taking the log of the magnitude is essentially what you do when you compute sound pressure level. Some define the cepstrum using the magnitude of the Fourier transform and some the magnitude squared. Squaring only introduces a multiple of 2 once we take logs, so it doesn't effect the location of peaks, only their amplitude.

Taking the logarithm compresses the peaks, bringing them all into roughly the same range, making the sequence of peaks roughly periodic.

When we take the inverse Fourier transform, we now have something like a frequency, but inverted. This is what Tukey called quefrency.

Looking at the guitar power spectrum above, we see a sequence of peaks spaced 440 Hz apart. When we take the inverse Fourier transform of this, we're looking at a sort of frequency of a frequency, what Tukey calls quefrency. The quefrency scale is inverted: sounds with a high frequency fundamental have overtones that are far apart on the frequency domain, so the sequence of the overtone peaks has low frequency.

Here's the plot of the cepstrum for the guitar sample.

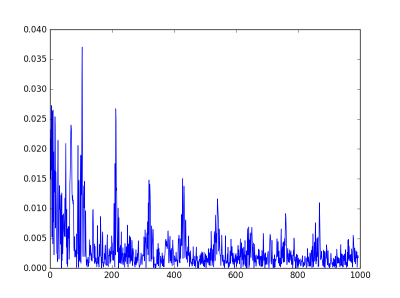
There's a big peak at 109 on the quefrency scale. The audio clip was recorded at 48000 samples per second, so the 109 on the quefrency scale corresponds to a frequency of 48000/109 = 440 Hz. The second peak is at quefrency 215, which corresponds to 48000/215 = 223 Hz. The second peak corresponds to the perceived pitch of the note, A3, and the first peak corresponds to its first harmonic, A4. (Remember the quefrency scale is inverted relative to the frequency scale.)

I cheated a little bit in the plot above. The very highest peaks are at 0. They are so large that they make it hard to see the peaks we're most interested in. These low quefrency peaks correspond to very high frequency noise, near the edge of the audible spectrum or beyond.