Exponential growth vs logistic growth
This seems like a good time to discuss the difference between exponential growth and logistic growth as the covid19 pandemic is starting to look more like a logistic model and less like an exponential model, at least in many parts of the world [1]. This post is an expansion of a Twitter thread I wrote on AnalysisFact.
***
Nothing in the world grows exponentially forever.
The beginning of exponential growth is easier to understand that its end.
From the beginning there are factors that keep growth from being purely exponential, but they are negligible at first. They can be ignored for a while, but not forever.
A logistic model takes one limiting factor into account, but things could be much more complicated than that.
Even though logistic models are often more realistic than exponential models, I wouldn't want someone to read this and say "Oh, I see. Epidemics (for example) aren't exponential, they're logistic." A logistic model is still a simple idealization, more realistic than an exponential model, but still simplistic.
A system with exponential growth satisfies
That is, the rate of growth y' at any given time is proportional to the state y. If you have money invested at a fixed rate of interest, for example, the amount of interest you earn is proportional to how much you've invested.
A system with logistic growth satisfies
That is, the rate of growth is the proportional to the product of two things: the current state y and the complement of the current state 1 - y. For example, a simple epidemic model says the rate of spread is proportional to the product of the proportion of infected people and the proportion of uninfected people. The rate of infection slows down as there are fewer people who have not been exposed.
(Let me say one more time that this is a simplistic model. Please don't read this then publish your own amateur analysis of a pandemic [2].)
Logistic growth applies to many things, not just epidemics. The spread of rumors, for example, is approximately logistic. The rate of spread depends on both the number of people spreading the rumor and the number of people who haven't heard. Once most people have heard, the rate slows way down because people are sharing the rumor with others who have already heard.
In the logistic differential equation above, when y is near 0, 1 - y is approximately 1 and so that term has little effect. That's why logistic models look like exponential models at first. Here are plots of exponential and logistic models with y(0) = 0.001, k = 0.1, and time running from 0 to 50.
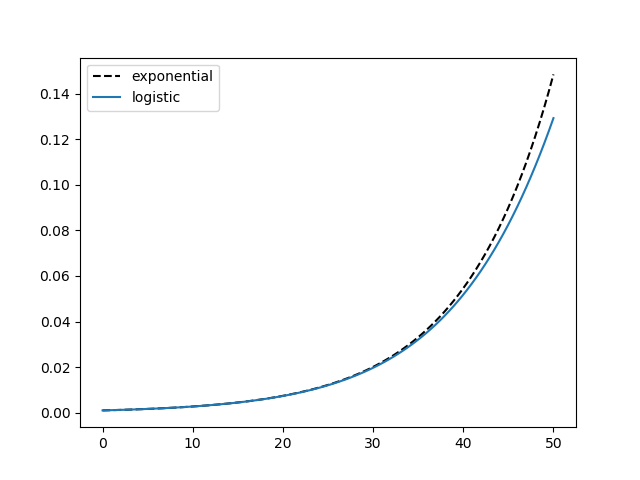
But the larger y gets, the more the 1 - y term matters more. Eventually it dominates. Here are the same functions for time running from 50 to 80.
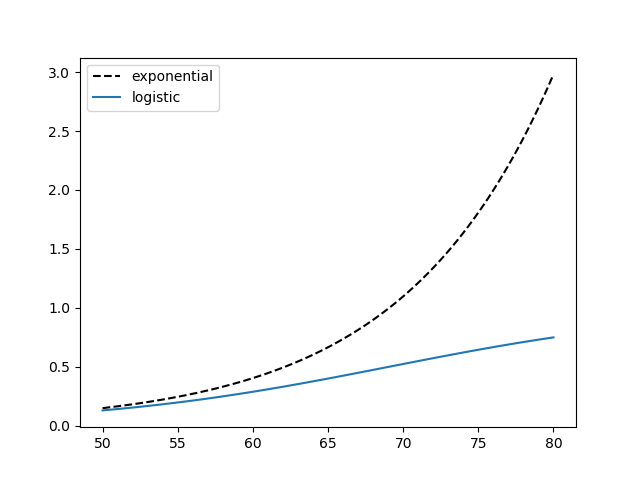
The logistic curve has a S shape and will flatten out, asymptotically approaching 1. Here's a comparison of the two plots with time running from 0 to 100.
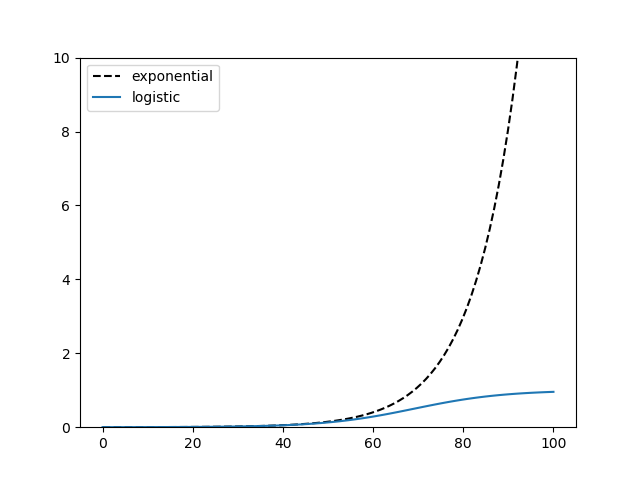
- Fixed points of the logistic function
- Sensitivity of logistic regression
- Approximating a logistic regression integral
[1] I recently heard from a friend in Niger that the country only had its first case on March 18, long after much of the world. Unfortunately the disease is spreading rapidly, and I imagine growing approximately exponentially for now. To make matters worse, the Niger is at the bottom of of the UN Heath Development Index as of 2018.
[2] Several people asked me from the beginning of the pandemic about mathematical models. I said that there would be many mathematical models, all over-simplified. I have not created or endorsed any model because I am not an epidemiologist. I have limited my comments to saying that epidemics are complex and there are a lot of unknowns.